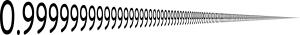Portal:Mathematics

Portal topics: Activities; Culture; Geography; Health; History; Mathematics; Nature; People; Philosophy; Religion; Society; Technology; Random portal

The Mathematics Portal

Mathematics is the study of representing and reasoning about abstract objects (such as numbers, points, spaces, sets, structures, and games). Mathematics is used throughout the world as an essential tool in many fields, including natural science, engineering, medicine, and the social sciences. Applied mathematics, the branch of mathematics concerned with application of mathematical knowledge to other fields, inspires and makes use of new mathematical discoveries and sometimes leads to the development of entirely new mathematical disciplines, such as statistics and game theory. Mathematicians also engage in pure mathematics, or mathematics for its own sake, without having any application in mind. There is no clear line separating pure and applied mathematics, and practical applications for what began as pure mathematics are often discovered. (Full article...)

Refresh with new selections below (purge)

Featured articles – load new batch

Featured articles are displayed here, which represent some of the best content on English Wikipedia.

Image 1

One of Molyneux's celestial globes, which is displayed in Middle Temple Library – from the frontispiece of the Hakluyt Society's 1889 reprint of A Learned Treatise of Globes, both Cœlestiall and Terrestriall, one of the English editions of Robert Hues' Latin work Tractatus de Globis (1594)

Emery Molyneux (/ˈɛməri ˈmɒlɪnoʊ/ EM-ər-ee MOL-in-oh; died June 1598) was an English Elizabethan maker of globes, mathematical instruments and ordnance. His terrestrial and celestial globes, first published in 1592, were the first to be made in England and the first to be made by an Englishman.

Molyneux was known as a mathematician and maker of mathematical instruments such as compasses and hourglasses. He became acquainted with many prominent men of the day, including the writer Richard Hakluyt and the mathematicians Robert Hues and Edward Wright. He also knew the explorers Thomas Cavendish, Francis Drake, Walter Raleigh and John Davis. Davis probably introduced Molyneux to his own patron, the London merchant William Sanderson, who largely financed the construction of the globes. When completed, the globes were presented to Elizabeth I. Larger globes were acquired by royalty, noblemen and academic institutions, while smaller ones were purchased as practical navigation aids for sailors and students. The globes were the first to be made in such a way that they were unaffected by the humidity at sea, and they came into general use on ships. (Full article...)
Image 2
Plots of logarithm functions, with three commonly used bases. The special points $log b b = 1$ are indicated by dotted lines, and all curves intersect in $log b 1 = 0$ .

In mathematics, the logarithm is the inverse function to exponentiation. That means that the logarithm of a number $x$ to the base $b$ is the exponent to which $b$ must be raised to produce $x$ . For example, since $1000 = 10 3$ , the logarithm base $10$ of $1000$ is $3$ , or $log 10 (1000) = 3$ . The logarithm of $x$ to base $b$ is denoted as $log b (x)$ , or without parentheses, $log b x$ . When the base is clear from the context or is irrelevant it is sometimes written $log x$ .

The logarithm base $10$ is called the decimal or common logarithm and is commonly used in science and engineering. The natural logarithm has the number $e \approx 2.718$ as its base; its use is widespread in mathematics and physics because of its very simple derivative. The binary logarithm uses base $2$ and is frequently used in computer science. (Full article...)
Image 3

Émile Michel Hyacinthe Lemoine (French: [emil ləmwan]; 22 November 1840 – 21 February 1912) was a French civil engineer and a mathematician, a geometer in particular. He was educated at a variety of institutions, including the Prytanée National Militaire and, most notably, the École Polytechnique. Lemoine taught as a private tutor for a short period after his graduation from the latter school.

Lemoine is best known for his proof of the existence of the Lemoine point (or the symmedian point) of a triangle. Other mathematical work includes a system he called Géométrographie and a method which related algebraic expressions to geometric objects. He has been called a co-founder of modern triangle geometry, as many of its characteristics are present in his work. (Full article...)
Image 4

Title page of the first edition of Wright's Certaine Errors in Navigation (1599)

Edward Wright (baptised 8 October 1561; died November 1615) was an English mathematician and cartographer noted for his book Certaine Errors in Navigation (1599; 2nd ed., 1610), which for the first time explained the mathematical basis of the Mercator projection by building on the works of Pedro Nunes, and set out a reference table giving the linear scale multiplication factor as a function of latitude, calculated for each minute of arc up to a latitude of 75°. This was in fact a table of values of the integral of the secant function, and was the essential step needed to make practical both the making and the navigational use of Mercator charts.

Wright was born at Garveston in Norfolk and educated at Gonville and Caius College, Cambridge, where he became a fellow from 1587 to 1596. In 1589 the college granted him leave after Elizabeth I requested that he carry out navigational studies with a raiding expedition organised by the Earl of Cumberland to the Azores to capture Spanish galleons. The expedition's route was the subject of the first map to be prepared according to Wright's projection, which was published in Certaine Errors in 1599. The same year, Wright created and published the first world map produced in England and the first to use the Mercator projection since Gerardus Mercator's original 1569 map. (Full article...)
$Image 5 The number π (/paɪ/; spelled out as "pi") is a mathematical constant that is the ratio of a circle's circumference to its diameter, approximately equal to 3.14159. The number π appears in many formulae across mathematics and physics. It is an irrational number, meaning that it cannot be expressed exactly as a ratio of two integers, although fractions such as '"`UNIQ--postMath-00000002-QINU`"' are commonly used to approximate it. Consequently, its decimal representation never ends, nor enters a permanently repeating pattern. It is a transcendental number, meaning that it cannot be a solution of an equation involving only finite sums, products, powers, and integers. The transcendence of π implies that it is impossible to solve the ancient challenge of squaring the circle with a compass and straightedge. The decimal digits of π appear to be randomly distributed, but no proof of this conjecture has been found. For thousands of years, mathematicians have attempted to extend their understanding of π, sometimes by computing its value to a high degree of accuracy. Ancient civilizations, including the Egyptians and Babylonians, required fairly accurate approximations of π for practical computations. Around 250 BC, the Greek mathematician Archimedes created an algorithm to approximate π with arbitrary accuracy. In the 5th century AD, Chinese mathematicians approximated π to seven digits, while Indian mathematicians made a five-digit approximation, both using geometrical techniques. The first computational formula for π, based on infinite series, was discovered a millennium later. The earliest known use of the Greek letter π to represent the ratio of a circle's circumference to its diameter was by the Welsh mathematician William Jones in 1706. (Full article...)$

Image 5
The number $π$ (/paɪ/; spelled out as "pi") is a mathematical constant that is the ratio of a circle's circumference to its diameter, approximately equal to 3.14159. The number $π$ appears in many formulae across mathematics and physics. It is an irrational number, meaning that it cannot be expressed exactly as a ratio of two integers, although fractions such as ${\tfrac {22}{7}}$ are commonly used to approximate it. Consequently, its decimal representation never ends, nor enters a permanently repeating pattern. It is a transcendental number, meaning that it cannot be a solution of an equation involving only finite sums, products, powers, and integers. The transcendence of $π$ implies that it is impossible to solve the ancient challenge of squaring the circle with a compass and straightedge. The decimal digits of $π$ appear to be randomly distributed, but no proof of this conjecture has been found.

For thousands of years, mathematicians have attempted to extend their understanding of $π$ , sometimes by computing its value to a high degree of accuracy. Ancient civilizations, including the Egyptians and Babylonians, required fairly accurate approximations of $π$ for practical computations. Around 250 BC, the Greek mathematician Archimedes created an algorithm to approximate $π$ with arbitrary accuracy. In the 5th century AD, Chinese mathematicians approximated $π$ to seven digits, while Indian mathematicians made a five-digit approximation, both using geometrical techniques. The first computational formula for $π$ , based on infinite series, was discovered a millennium later. The earliest known use of the Greek letter π to represent the ratio of a circle's circumference to its diameter was by the Welsh mathematician William Jones in 1706. (Full article...)
Image 6
General relativity, also known as the general theory of relativity, and as Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. General relativity generalizes special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time or four-dimensional spacetime. In particular, the curvature of spacetime is directly related to the energy and momentum of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of second-order partial differential equations.

Newton's law of universal gravitation, which describes classical gravity, can be seen as a prediction of general relativity for the almost flat spacetime geometry around stationary mass distributions. Some predictions of general relativity, however, are beyond Newton's law of universal gravitation in classical physics. These predictions concern the passage of time, the geometry of space, the motion of bodies in free fall, and the propagation of light, and include gravitational time dilation, gravitational lensing, the gravitational redshift of light, the Shapiro time delay and singularities/black holes. So far, all tests of general relativity have been shown to be in agreement with the theory. The time-dependent solutions of general relativity enable us to talk about the history of the universe and have provided the modern framework for cosmology, thus leading to the discovery of the Big Bang and cosmic microwave background radiation. Despite the introduction of a number of alternative theories, general relativity continues to be the simplest theory consistent with experimental data. (Full article...)
Image 7

Damage from Hurricane Katrina in 2005. Actuaries need to estimate long-term levels of such damage in order to accurately price property insurance, set appropriate reserves, and design appropriate reinsurance and capital management strategies.

An actuary is a professional with advanced mathematical skills who deals with the measurement and management of risk and uncertainty. The name of the corresponding field is actuarial science which covers rigorous mathematical calculations in areas of life expectancy and life insurance. These risks can affect both sides of the balance sheet and require asset management, liability management, and valuation skills. Actuaries provide assessments of financial security systems, with a focus on their complexity, their mathematics, and their mechanisms.

While the concept of insurance dates to antiquity, the concepts needed to scientifically measure and mitigate risks have their origins in the 17th century studies of probability and annuities. Actuaries of the 21st century require analytical skills, business knowledge, and an understanding of human behavior and information systems to design and manage programs that control risk. The actual steps needed to become an actuary are usually country-specific; however, almost all processes share a rigorous schooling or examination structure and take many years to complete. (Full article...)
Image 8

Hilary Putnam

The Quine–Putnam indispensability argument is an argument in the philosophy of mathematics for the existence of abstract mathematical objects such as numbers and sets, a position known as mathematical platonism. It was named after the philosophers Willard Quine and Hilary Putnam, and is one of the most important arguments in the philosophy of mathematics.

Although elements of the indispensability argument may have originated with thinkers such as Gottlob Frege and Kurt Gödel, Quine's development of the argument was unique for introducing to it a number of his philosophical positions such as naturalism, confirmational holism, and the criterion of ontological commitment. Putnam gave Quine's argument its first detailed formulation in his 1971 book Philosophy of Logic. He later came to disagree with various aspects of Quine's thinking, however, and formulated his own indispensability argument based on the no miracles argument in the philosophy of science. A standard form of the argument in contemporary philosophy is credited to Mark Colyvan; whilst being influenced by both Quine and Putnam, it differs in important ways from their formulations. It is presented in the Stanford Encyclopedia of Philosophy: (Full article...)
Image 9
Figure 1: A solution (in purple) to Apollonius's problem. The given circles are shown in black.

In Euclidean plane geometry, Apollonius's problem is to construct circles that are tangent to three given circles in a plane (Figure 1). Apollonius of Perga (c. 262 BC – c. 190 BC) posed and solved this famous problem in his work Ἐπαφαί (Epaphaí, "Tangencies"); this work has been lost, but a 4th-century AD report of his results by Pappus of Alexandria has survived. Three given circles generically have eight different circles that are tangent to them (Figure 2), a pair of solutions for each way to divide the three given circles in two subsets (there are 4 ways to divide a set of cardinality 3 in 2 parts).

In the 16th century, Adriaan van Roomen solved the problem using intersecting hyperbolas, but this solution does not use only straightedge and compass constructions. François Viète found such a solution by exploiting limiting cases: any of the three given circles can be shrunk to zero radius (a point) or expanded to infinite radius (a line). Viète's approach, which uses simpler limiting cases to solve more complicated ones, is considered a plausible reconstruction of Apollonius' method. The method of van Roomen was simplified by Isaac Newton, who showed that Apollonius' problem is equivalent to finding a position from the differences of its distances to three known points. This has applications in navigation and positioning systems such as LORAN. (Full article...)
Image 10
High-precision test of general relativity by the Cassini space probe (artist's impression): radio signals sent between the Earth and the probe (green wave) are delayed by the warping of spacetime (blue lines) due to the Sun's mass.

General relativity is a theory of gravitation developed by Albert Einstein between 1907 and 1915. The theory of general relativity says that the observed gravitational effect between masses results from their warping of spacetime.

By the beginning of the 20th century, Newton's law of universal gravitation had been accepted for more than two hundred years as a valid description of the gravitational force between masses. In Newton's model, gravity is the result of an attractive force between massive objects. Although even Newton was troubled by the unknown nature of that force, the basic framework was extremely successful at describing motion. (Full article...)
Image 11

Archimedes Thoughtful
by Domenico Fetti (1620)

Archimedes of Syracuse (/ˌɑːrkɪˈmiːdiːz/ AR-kim-EE-deez; c. 287 – c. 212 BC) was an Ancient Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists in classical antiquity. Considered the greatest mathematician of ancient history, and one of the greatest of all time, Archimedes anticipated modern calculus and analysis by applying the concept of the infinitely small and the method of exhaustion to derive and rigorously prove a range of geometrical theorems. These include the area of a circle, the surface area and volume of a sphere, the area of an ellipse, the area under a parabola, the volume of a segment of a paraboloid of revolution, the volume of a segment of a hyperboloid of revolution, and the area of a spiral.

Archimedes' other mathematical achievements include deriving an approximation of pi, defining and investigating the Archimedean spiral, and devising a system using exponentiation for expressing very large numbers. He was also one of the first to apply mathematics to physical phenomena, working on statics and hydrostatics. Archimedes' achievements in this area include a proof of the law of the lever, the widespread use of the concept of center of gravity, and the enunciation of the law of buoyancy known as Archimedes' principle. He is also credited with designing innovative machines, such as his screw pump, compound pulleys, and defensive war machines to protect his native Syracuse from invasion. (Full article...)
Image 12

Portrait by Jakob Emanuel Handmann, 1753

Leonhard Euler (/ˈɔɪlər/ OY-lər, German: [ˈleːɔnhaʁt ˈʔɔʏlɐ] , Swiss Standard German: [ˈleːɔnhart ˈɔʏlər]; 15 April 1707 – 18 September 1783) was a Swiss mathematician, physicist, astronomer, geographer, logician, and engineer who founded the studies of graph theory and topology and made pioneering and influential discoveries in many other branches of mathematics such as analytic number theory, complex analysis, and infinitesimal calculus. He introduced much of modern mathematical terminology and notation, including the notion of a mathematical function. He is also known for his work in mechanics, fluid dynamics, optics, astronomy, and music theory.

Euler is held to be one of the greatest, most prolific mathematicians in history and the greatest of the 18th century. Several great mathematicians who produced their work after Euler's death have recognised his importance in the field as shown by quotes attributed to many of them: Pierre-Simon Laplace expressed Euler's influence on mathematics by stating, "Read Euler, read Euler, he is the master of us all." Carl Friedrich Gauss wrote: "The study of Euler's works will remain the best school for the different fields of mathematics, and nothing else can replace it." His 866 publications as well as his correspondences are being collected in the Opera Omnia Leonhard Euler which, when completed, will consist of 81 quartos. He spent most of his adult life in Saint Petersburg, Russia, and in Berlin, then the capital of Prussia. (Full article...)
Image 13
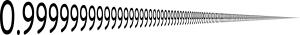
Stylistic impression of the number, representing how its decimals go on infinitely

In mathematics, 0.999... (also written as 0.9, 0..9, or 0.(9)) denotes the smallest number greater than every number in the sequence (0.9, 0.99, 0.999, ...). It can be proved that this number is 1; that is,
: $0.999...=1.$
In other words, 0.999... is not "almost exactly 1" or "very, very nearly but not quite 1"; rather, 0.999... and "1" are exactly the same number.

An elementary proof is given below that involves only elementary arithmetic and the fact that there is no positive real number less than all 1/10^$n$, where $n$ is a natural number, a property that results immediately from the Archimedean property of the real numbers. (Full article...)
Image 14

Portrait by August Köhler, c. 1910, after 1627 original

Johannes Kepler (/ˈkɛplər/; German: [joˈhanəs ˈkɛplɐ, -nɛs -] ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws of planetary motion, and his books Astronomia nova, Harmonice Mundi, and Epitome Astronomiae Copernicanae, influencing among others Isaac Newton, providing one of the foundations for his theory of universal gravitation. The variety and impact of his work made Kepler one of the founders and fathers of modern astronomy, the scientific method, natural and modern science. He has been described as the "father of science fiction" for his novel Somnium.

Kepler was a mathematics teacher at a seminary school in Graz, where he became an associate of Prince Hans Ulrich von Eggenberg. Later he became an assistant to the astronomer Tycho Brahe in Prague, and eventually the imperial mathematician to Emperor Rudolf II and his two successors Matthias and Ferdinand II. He also taught mathematics in Linz, and was an adviser to General Wallenstein.
Additionally, he did fundamental work in the field of optics, being named the father of modern optics, in particular for his Astronomiae pars optica. He also invented an improved version of the refracting telescope, the Keplerian telescope, which became the foundation of the modern refracting telescope, while also improving on the telescope design by Galileo Galilei, who mentioned Kepler's discoveries in his work. (Full article...)
Image 15
In algebraic geometry and theoretical physics, mirror symmetry is a relationship between geometric objects called Calabi–Yau manifolds. The term refers to a situation where two Calabi–Yau manifolds look very different geometrically but are nevertheless equivalent when employed as extra dimensions of string theory.

Early cases of mirror symmetry were discovered by physicists. Mathematicians became interested in this relationship around 1990 when Philip Candelas, Xenia de la Ossa, Paul Green, and Linda Parkes showed that it could be used as a tool in enumerative geometry, a branch of mathematics concerned with counting the number of solutions to geometric questions. Candelas and his collaborators showed that mirror symmetry could be used to count rational curves on a Calabi–Yau manifold, thus solving a longstanding problem. Although the original approach to mirror symmetry was based on physical ideas that were not understood in a mathematically precise way, some of its mathematical predictions have since been proven rigorously. (Full article...)

More featured articles

edit

Selected image – show another

Line integral

Credit: Lucas V. Barbosa

A line integral is an integral where the function to be integrated, be it a scalar field as here or a vector field, is evaluated along a curve. The value of the line integral is the sum of values of the field at all points on the curve, weighted by some scalar function on the curve (commonly arc length or, for a vector field, the scalar product of the vector field with a differential vector in the curve). A detailed explanation of the animation is available. The key insight is that line integrals may be reduced to simpler definite integrals. (See also a similar animation illustrating a line integral of a vector field.) Many formulas in elementary physics (for example,

W = F \cdot s

to find the work done by a constant force

F

in moving an object through a displacement

s

) have line integral versions that work for non-constant quantities (for example,

W = \int C F \cdot d s

to find the work done in moving an object along a curve C within a continuously varying gravitational or electric field

F

). A higher-dimensional analog of a line integral is a surface integral, where the (double) integral is taken over a two-dimensional surface instead of along a one-dimensional curve. Surface integrals can also be thought of as generalizations of multiple integrals. All of these can be seen as special cases of integrating a differential form, a viewpoint which allows multivariable calculus to be done independently of the choice of coordinate system. While the elementary notions upon which integration is based date back centuries before Newton and Leibniz independently invented calculus, line and surface integrals were formalized in the 18th and 19th centuries as the subject was placed on a rigorous mathematical foundation. The modern notion of differential forms, used extensively in differential geometry and quantum physics, was pioneered by Élie Cartan in the late 19th century.

More selected pictures

Good articles – load new batch

These are Good articles, which meet a core set of high editorial standards.

Image 1
The Laves graph

In geometry and crystallography, the Laves graph is an infinite and highly symmetric system of points and line segments in three-dimensional Euclidean space, forming a periodic graph. Three equal-length segments meet at 120° angles at each point, and all cycles use ten or more segments. It is the shortest possible triply periodic graph, relative to the volume of its fundamental domain. One arrangement of the Laves graph uses one out of every eight of the points in the integer lattice as its points, and connects all pairs of these points that are nearest neighbors, at distance ${\sqrt {2}}$ . It can also be defined, divorced from its geometry, as an abstract undirected graph, a covering graph of the complete graph on four vertices.
H. S. M. Coxeter (1955) named this graph after Fritz Laves, who first wrote about it as a crystal structure in 1932. It has also been called the K₄ crystal, (10,3)-a network, diamond twin, triamond, and the srs net. The regions of space nearest each vertex of the graph are congruent 17-sided polyhedra that tile space. Its edges lie on diagonals of the regular skew polyhedron, a surface with six squares meeting at each integer point of space. (Full article...)
Image 2
Lighting and reflection calculations, as in the video game OpenArena, use the fast inverse square root code to compute angles of incidence and reflection.

Fast inverse square root, sometimes referred to as Fast InvSqrt() or by the hexadecimal constant 0x5F3759DF, is an algorithm that estimates ${\textstyle {\frac {1}{\sqrt {x}}}}$ , the reciprocal (or multiplicative inverse) of the square root of a 32-bit floating-point number $x$ in IEEE 754 floating-point format. The algorithm is best known for its implementation in 1999 in Quake III Arena, a first-person shooter video game heavily based on 3D graphics. With subsequent hardware advancements, especially the x86 SSE instruction rsqrtss, this algorithm is not generally the best choice for modern computers, though it remains an interesting historical example.

The algorithm accepts a 32-bit floating-point number as the input and stores a halved value for later use. Then, treating the bits representing the floating-point number as a 32-bit integer, a logical shift right by one bit is performed and the result subtracted from the number 0x5F3759DF, which is a floating-point representation of an approximation of ${\textstyle {\sqrt {2^{127}}}}$ . This results in the first approximation of the inverse square root of the input. Treating the bits again as a floating-point number, it runs one iteration of Newton's method, yielding a more precise approximation. (Full article...)
$Image 3 Graphical demonstration of the convergence of the sum 1/2 + 1/3 + 1/7 + 1/43 + ... to 1. Each row of k squares of side length 1/k has total area 1/k, and all the squares together exactly cover a larger square with area 1. Squares with side lengths 1/1807 or smaller are too small to see in the figure and are not shown. In number theory, Sylvester's sequence is an integer sequence in which each term is the product of the previous terms, plus one. Its first few terms are :2, 3, 7, 43, 1807, 3263443, 10650056950807, 113423713055421844361000443 (sequence A000058 in the OEIS). Sylvester's sequence is named after James Joseph Sylvester, who first investigated it in 1880. Its values grow doubly exponentially, and the sum of its reciprocals forms a series of unit fractions that converges to 1 more rapidly than any other series of unit fractions. The recurrence by which it is defined allows the numbers in the sequence to be factored more easily than other numbers of the same magnitude, but, due to the rapid growth of the sequence, complete prime factorizations are known only for a few of its terms. Values derived from this sequence have also been used to construct finite Egyptian fraction representations of 1, Sasakian Einstein manifolds, and hard instances for online algorithms.[1] (Full article...)$

Image 3
Graphical demonstration of the convergence of the sum 1/2 + 1/3 + 1/7 + 1/43 + ... to 1. Each row of k squares of side length 1/k has total area 1/k, and all the squares together exactly cover a larger square with area 1. Squares with side lengths 1/1807 or smaller are too small to see in the figure and are not shown.

In number theory, Sylvester's sequence is an integer sequence in which each term is the product of the previous terms, plus one. Its first few terms are
:2, 3, 7, 43, 1807, 3263443, 10650056950807, 113423713055421844361000443 (sequence A000058 in the OEIS).

Sylvester's sequence is named after James Joseph Sylvester, who first investigated it in 1880. Its values grow doubly exponentially, and the sum of its reciprocals forms a series of unit fractions that converges to 1 more rapidly than any other series of unit fractions. The recurrence by which it is defined allows the numbers in the sequence to be factored more easily than other numbers of the same magnitude, but, due to the rapid growth of the sequence, complete prime factorizations are known only for a few of its terms. Values derived from this sequence have also been used to construct finite Egyptian fraction representations of 1, Sasakian Einstein manifolds, and hard instances for online algorithms.^[1] (Full article...)
Image 4

Francis Amasa Walker

Francis Amasa Walker (July 2, 1840 – January 5, 1897) was an American economist, statistician, journalist, educator, academic administrator, and an officer in the Union Army.

Walker was born into a prominent Boston family, the son of the economist and politician Amasa Walker, and he graduated from Amherst College at the age of 20. He received a commission to join the 15th Massachusetts Infantry and quickly rose through the ranks as an assistant adjutant general. Walker fought in the Peninsula, Bristoe, Overland, and Richmond-Petersburg Campaigns before being captured by Confederate forces and held at the infamous Libby Prison. In July 1866, he was awarded the honorary grade of brevet brigadier general United States Volunteers, to rank from March 13, 1865, when he was 24 years old. (Full article...)
Image 5

Roman copy (in marble) of a Greek bronze bust of Aristotle by Lysippos (c. 330 BC), with modern alabaster mantle

Aristotle (Greek: Ἀριστοτέλης Aristotélēs; 384–322 BC) was an Ancient Greek philosopher and polymath. His writings cover a broad range of subjects spanning the natural sciences, philosophy, linguistics, economics, politics, psychology, and the arts. As the founder of the Peripatetic school of philosophy in the Lyceum in Athens, he began the wider Aristotelian tradition that followed, which set the groundwork for the development of modern science.

Little is known about Aristotle's life. He was born in the city of Stagira in northern Greece during the Classical period. His father, Nicomachus, died when Aristotle was a child, and he was brought up by a guardian. At 17 or 18, he joined Plato's Academy in Athens and remained there until the age of 37 (c. 347 BC). Shortly after Plato died, Aristotle left Athens and, at the request of Philip II of Macedon, tutored his son Alexander the Great beginning in 343 BC. He established a library in the Lyceum, which helped him to produce many of his hundreds of books on papyrus scrolls. (Full article...)
Image 6
In geometry, the Beckman–Quarles theorem states that if a transformation of the Euclidean plane or a higher-dimensional Euclidean space preserves unit distances, then it preserves all Euclidean distances. Equivalently, every homomorphism from the unit distance graph of the plane to itself must be an isometry of the plane. The theorem is named after Frank S. Beckman and Donald A. Quarles Jr., who published this result in 1953; it was later rediscovered by other authors and re-proved in multiple ways. Analogous theorems for rational subsets of Euclidean spaces, or for non-Euclidean geometry, are also known. (Full article...)
Image 7
An $m \times n$ matrix: the $m$ rows are horizontal and the $n$ columns are vertical. Each element of a matrix is often denoted by a variable with two subscripts. For example, $a 2,1$ represents the element at the second row and first column of the matrix.

In mathematics, a matrix (pl.: matrices) is a rectangular array or table of numbers, symbols, or expressions, with elements or entries arranged in rows and columns, which is used to represent a mathematical object or property of such an object.

For example,
${\begin{bmatrix}1&9&-13\\20&5&-6\end{bmatrix}}$
is a matrix with two rows and three columns. This is often referred to as a "two-by-three matrix", a " $2\times 3$ matrix", or a matrix of dimension $2\times 3$ . (Full article...)
$Image 8 Slices of approximately 1/8 of a pizza A unit fraction is a positive fraction with one as its numerator, 1/n. It is the multiplicative inverse (reciprocal) of the denominator of the fraction, which must be a positive natural number. Examples are 1/1, 1/2, 1/3, 1/4, 1/5, etc. When an object is divided into equal parts, each part is a unit fraction of the whole. Multiplying two unit fractions produces another unit fraction, but other arithmetic operations do not preserve unit fractions. In modular arithmetic, unit fractions can be converted into equivalent whole numbers, allowing modular division to be transformed into multiplication. Every rational number can be represented as a sum of distinct unit fractions; these representations are called Egyptian fractions based on their use in ancient Egyptian mathematics. Many infinite sums of unit fractions are meaningful mathematically. (Full article...)$

Image 8
Slices of approximately 1/8 of a pizza

A unit fraction is a positive fraction with one as its numerator, 1/ $n$ . It is the multiplicative inverse (reciprocal) of the denominator of the fraction, which must be a positive natural number. Examples are 1/1, 1/2, 1/3, 1/4, 1/5, etc. When an object is divided into equal parts, each part is a unit fraction of the whole.

Multiplying two unit fractions produces another unit fraction, but other arithmetic operations do not preserve unit fractions. In modular arithmetic, unit fractions can be converted into equivalent whole numbers, allowing modular division to be transformed into multiplication. Every rational number can be represented as a sum of distinct unit fractions; these representations are called Egyptian fractions based on their use in ancient Egyptian mathematics. Many infinite sums of unit fractions are meaningful mathematically. (Full article...)
Image 9
Wave functions of the electron in a hydrogen atom at different energy levels. Quantum mechanics cannot predict the exact location of a particle in space, only the probability of finding it at different locations. The brighter areas represent a higher probability of finding the electron.

Quantum mechanics is a fundamental theory that describes the behavior of nature at and below the scale of atoms. It is the foundation of all quantum physics, which includes quantum chemistry, quantum field theory, quantum technology, and quantum information science.

Quantum mechanics can describe many systems that classical physics cannot. Classical physics can describe many aspects of nature at an ordinary (macroscopic and (optical) microscopic) scale, but is not sufficient for describing them at very small submicroscopic (atomic and subatomic) scales. Most theories in classical physics can be derived from quantum mechanics as an approximation valid at large (macroscopic/microscopic) scale. (Full article...)
Image 10
The cover page of Ars Conjectandi

Ars Conjectandi (Latin for "The Art of Conjecturing") is a book on combinatorics and mathematical probability written by Jacob Bernoulli and published in 1713, eight years after his death, by his nephew, Niklaus Bernoulli. The seminal work consolidated, apart from many combinatorial topics, many central ideas in probability theory, such as the very first version of the law of large numbers: indeed, it is widely regarded as the founding work of that subject. It also addressed problems that today are classified in the twelvefold way and added to the subjects; consequently, it has been dubbed an important historical landmark in not only probability but all combinatorics by a plethora of mathematical historians. The importance of this early work had a large impact on both contemporary and later mathematicians; for example, Abraham de Moivre.

Bernoulli wrote the text between 1684 and 1689, including the work of mathematicians such as Christiaan Huygens, Gerolamo Cardano, Pierre de Fermat, and Blaise Pascal. He incorporated fundamental combinatorial topics such as his theory of permutations and combinations (the aforementioned problems from the twelvefold way) as well as those more distantly connected to the burgeoning subject: the derivation and properties of the eponymous Bernoulli numbers, for instance. Core topics from probability, such as expected value, were also a significant portion of this important work. (Full article...)
Image 11
In mathematics, the factorial of a non-negative integer $n$ , denoted by $n!$ , is the product of all positive integers less than or equal to $n$ . The factorial of $n$ also equals the product of $n$ with the next smaller factorial:
$<br>{\begin{aligned}n!&=n\times (n-1)\times (n-2)\times (n-3)\times \cdots \times 3\times 2\times 1\\<br>&=n\times (n-1)!\\<br>\end{aligned}}$
For example,
$5!=5\times 4!=5\times 4\times 3\times 2\times 1=120.$
The value of 0! is 1, according to the convention for an empty product.

Factorials have been discovered in several ancient cultures, notably in Indian mathematics in the canonical works of Jain literature, and by Jewish mystics in the Talmudic book Sefer Yetzirah. The factorial operation is encountered in many areas of mathematics, notably in combinatorics, where its most basic use counts the possible distinct sequences – the permutations – of $n$ distinct objects: there are $n!$ . In mathematical analysis, factorials are used in power series for the exponential function and other functions, and they also have applications in algebra, number theory, probability theory, and computer science. (Full article...)
Image 12
Schwarz lantern on display in the German Museum of Technology, Berlin

In mathematics, the Schwarz lantern is a polyhedral approximation to a cylinder, used as a pathological example of the difficulty of defining the area of a smooth (curved) surface as the limit of the areas of polyhedra. It is formed by stacked rings of isosceles triangles, arranged within each ring in the same pattern as an antiprism. The resulting shape can be folded from paper, and is named after mathematician Hermann Schwarz and for its resemblance to a cylindrical paper lantern. It is also known as Schwarz's boot, Schwarz's polyhedron, or the Chinese lantern.

As Schwarz showed, for the surface area of a polyhedron to converge to the surface area of a curved surface, it is not sufficient to simply increase the number of rings and the number of isosceles triangles per ring. Depending on the relation of the number of rings to the number of triangles per ring, the area of the lantern can converge to the area of the cylinder, to a limit arbitrarily larger than the area of the cylinder, or to infinity—in other words, the area can diverge. The Schwarz lantern demonstrates that sampling a curved surface by close-together points and connecting them by small triangles is inadequate to ensure an accurate approximation of area, in contrast to the accurate approximation of arc length by inscribed polygonal chains. (Full article...)

More good articles

Did you know (auto-generated) – load new batch

... that the discovery of Descartes' theorem in geometry came from a too-difficult mathematics problem posed to a princess?
... that owner Matthew Benham influenced both Brentford FC in the UK and FC Midtjylland in Denmark to use mathematical modelling to recruit undervalued football players?
... that the word algebra is derived from an Arabic term for the surgical treatment of bonesetting?
... that in 1940 Xu Ruiyun became the first Chinese woman to receive a PhD in mathematics?
... that Fairleigh Dickinson's upset victory over Purdue was the biggest upset in terms of point spread in NCAA tournament history, with Purdue being a 23+1⁄2-point favorite?
... that two members of the French parliament were killed when a delayed-action German bomb exploded in the town hall at Bapaume on 25 March 1917?
... that in the aftermath of the American Civil War, the only Black-led organization providing teachers to formerly enslaved people was the African Civilization Society?
... that after Florida schools banned 54 mathematics books, Chaz Stevens petitioned that they also ban the Bible?

edit

More did you know – view different entries

Did you know...

...that the Catalan numbers solve a number of problems in combinatorics such as the number of ways to completely parenthesize an algebraic expression with n+1 factors?
...that a ball can be cut up and reassembled into two balls, each the same size as the original (Banach-Tarski paradox)?
...that it is impossible to devise a single formula involving only polynomials and radicals for solving an arbitrary quintic equation?
...that Euler found 59 more amicable numbers while for 2000 years, only 3 pairs had been found before him?
...that you cannot knot strings in 4 dimensions, but you can knot 2-dimensional surfaces, such as spheres?
...that there are 6 unsolved mathematics problems whose solutions will earn you one million US dollars each?
...that there are different sizes of infinite sets in set theory? More precisely, not all infinite cardinal numbers are equal?

More did you know facts

Showing 7 items out of 75

edit

Selected article – show another

Fractals arise in surprising places, in this case, the famous Collatz conjecture in number theory.
Image credit: Pokipsy76

A fractal is "a rough or fragmented geometric shape that can be subdivided in parts, each of which is (at least approximately) a reduced-size copy of the whole". The term was coined by Benoît Mandelbrot in 1975 and was derived from the Latin fractus meaning "broken" or "fractured".

A fractal as a geometric object generally has the following features:

It has a fine structure at arbitrarily small scales.
It is too irregular to be easily described in traditional Euclidean geometric language.
It is self-similar (at least approximately or stochastically).
It has a Hausdorff dimension which is greater than its topological dimension (although this requirement is not met by space-filling curves such as the Hilbert curve).
It has a simple and recursive definition.

Because they appear similar at all levels of magnification, fractals are often considered to be infinitely complex (in informal terms). Natural objects that approximate fractals to a degree include clouds, mountain ranges, lightning bolts, coastlines, and snow flakes. However, not all self-similar objects are fractals—for example, the real line (a straight Euclidean line) is formally self-similar but fails to have other fractal characteristics. Fractals, when zoomed in, will keep showing more and more of itself, and it keeps going for infinity. (Full article...)

View all selected articles

edit

Subcategories

Full category tree. Select [►] to view subcategories.

More mathematics categories

edit

Topics in mathematics

General

Foundations

Number theory

Discrete mathematics

Algebra

Analysis

Geometry and topology

Applied mathematics

edit

Index of mathematics articles

ARTICLE INDEX:	0–9 A B C D E F G H I J K L M N O P Q R S T U V W X Y Z
MATHEMATICIANS:	A B C D E F G H I J K L M N O P Q R S T U V W X Y Z